Quantum vortex and quantum turbulence
|
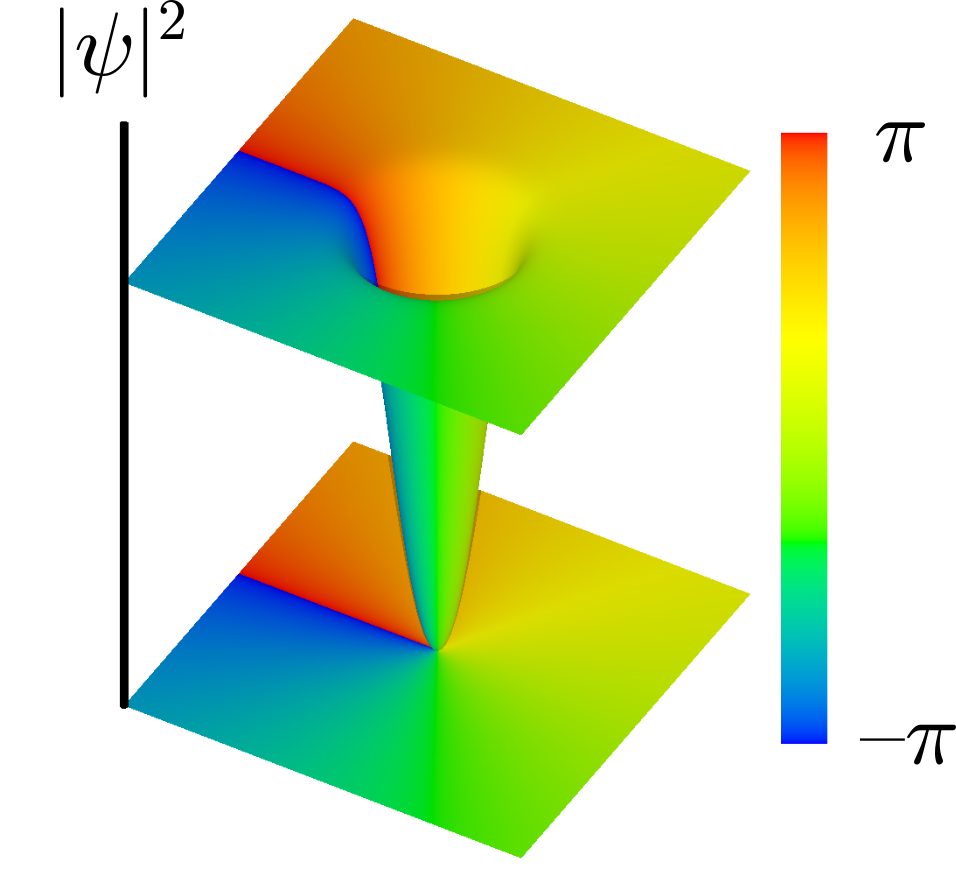
Quantum fluid such as superconductors and superfluids shows quantum effects in macroscopic scales, realized at low temperatures and inducing dissipationless flows (superconductors: zero resistance, superfluid: zero viscosity). The nature of dissipationless flow is the complex field \( \psi(\boldsymbol{x},t) \) called macroscopic wave function. Denoting the complex field with the amplitude and the phase: \( \psi(\boldsymbol{x},t) = |\psi(\boldsymbol{x},t)| e^{i \phi(\boldsymbol{x},t)} \), we can obtain the flow field \( \boldsymbol{v}(\boldsymbol{x},t) \) as the gradient of the phase \( \phi \): \( \boldsymbol{v}(\boldsymbol{x},t) = (\hbar / m) \nabla \phi \) (\( \hbar \) is the Planck constant and \( m \) the particle mass constituting quantum fluid). Flow becomes irrotational except for a point with a quantized vortex which is a topological defect (the amplitude vanishes and the phase rotate by \( 2 \pi \)). Being different from vortices in conventional viscous fluid, the quantized vortex have a constant flow strength (vorticity). A typical structure of the complex field with a quantized vortex is shown in the left figure. |
|
|
What happens for rotating quantum fluid? Because a quantized vortex has a fixed vorticity unlike that in conventional fluid having arbitrary vorticity, vortices enter from the edge of the container and form a vortex lattice. Showing in a right figure is the simulation of rotating quantum fluid in a container having a rough surface. |
Vortex dynamics in a rotating container. |
|
Turbulent state in quantum fluid is called quantum turbulence (or superfluid turbulence). As well as turbulence in conventional fluid (classical turbulence), it has strongly fluctuating fluid structure in both space and time, and vortices have a highly complicated tangling dynamics. Showing in a right figure is a vortex dynamics in quantum turbulence. Not only recognizing turbulence as complicated flow structure, there are but also many challenges to find some law in turbulence since Leonardo Da Vinci. One of the most important laws for turbulence is the Kolmogorov's statistical law for fully developed turbulence. Recently, it has been observed that the Kolmogorov's law is also satisfied in quantum turbulence. This result suggests some universal structure in turbulence not restricted to classical or quantum turbulence. Furthermore, a quantized vortex has the defined structure as the topological defect, there are some challenges to find a universal vortex dynamics in turbulence by analysing quantum turbulence. Recently, some statistical laws characteristic of quantum turbulence have also been found. |
Vortex dynamics in quantum turbulence. |
Abelian and non-Abelian vortices
|
Quantized vortex can have a fixed vorticity as said in above. More precisely, quantized vortex can be classified by discrete values (like energy eigenvalues in the Schrödinger equation).
In superfluid helium, the vorticity (line integral of the velocity around the vortex) is the integer multiple of \( 2 \pi \hbar / m \), i.e., \( 2 \pi \hbar n / m \) (\( n \in \mathbb{Z} \)) (However, a vortex with \( |n| \geq 2 \) is unstable and usually splits into vortices with \( |n| = 1 \)). In this case an integer \( n \) classifies the quantized vortex, called a topological charge of the quantized vortex. However, the topological charge sometimes becomes not a scalar but a matrix when particles constituting quantum fluid have internal degrees of freedom such as the orbital or spin angular momentum. The multiplication of two matrices is non-commutative in general. Quantized vortices having commutative and non-commutative topological charges are called Abelian and non-Abelian vortices respectively. Because topological charges of quantized vortices in superfluid helium is not matrices but integers, quantized vortices are Abelian. The difference between Abelian and non-Abelian vortices emerges in collision dynamics of two vortices. Showing in a right movie 1 is a collision dynamics of two Abelian vortices. After colliding each other, they reconnect changing the topology. Abelian vortices sometimes pass through each other as showing in a right movie 2 instead of reconnecting. |
Reconnection (movie 1) and passing through (movie 2) of Abelian vortices. |
| Reconnection and passing through are topologically forbidden for non-Abelian vortices. As a result, a new vortex (we call rung vortex) appears between colliding two vortices, bridging them. The topological charge of the rung vortex is determined by the commutation relation between topological charges of colliding vortices. Showing in right movies 1 and 2 is collision dynamics of non-Abelian vortices. Because non-Abelian properties are different between two movies (same conjugacy class in the movie 1 and different conjugacy class in the movie 2), there is a little difference between the two movies. | Dynamics of a formation of a rung vortex (movie 1: same conjugacy class, movie 2: different conjugacy class). |
Phase transition induced by quantized vortices
|
A quantized vortex takes the line structure in the 3-dimensional system and the point structure in the 2-dimensional system such as thin films. In 2-dimensional system,it is prohibited for a spontaneous breaking of the continuous symmetry such as a phase transition from fluid to crystal to occur at finite temperatures. However, a unique phase transition called Berezinskii-Kosterlitz-Thouless (BKT) transition can occur when the system can carry point-like topological defects such as quantized vortices, which are topologically stable defects and cannot be annihilated through a continuous change of a wave function \( \psi \to \psi + \psi^\prime \). A vortex can be annihilated as a pair with its "anti" defect having opposite flow. At finite temperatures, a quantized vortex is nucleated and annihilated as a pair of vortex and anti-vortex. At low temperatures, a vortex is nucleated with its anti-vortex and annihilated soon with the same anti-vortex (right movie-1).At high temperatures, however, a vortex sometimes survives without be annihilated with the original anti-vortex and moves as Brownian motion (right movie-2). There is a definite temperature called BKT transition temperature dividing these two motions of quantized vortices. At lower temperature below the BKT transition temperature, the system can carry a inviscid superflow. A simple BKT transition have long studied with, for example, XY ferromagnets. We are now studying complicated situations such as the relationship between topological charges of quantized vortices and the BKT transition. For example, it has not been well understood about what happens for the BKT transition with non-Abelian quantized vortices. |
Vortex motions at the temperature lower thant BKT transition temperature (movie-1) and higher BKT transition temperature (movie-2). Blue and red points show the position of vortices and anti-vortices. |
Nambu-Goldstone mode localized on a topological defect
|
When a continuous symmetry is spontaneously broken with a phase transition, a Nambu-Goldstone (NG) mode appears. For example, the Heisenberg spin model for the 3-dimensional spin shows the spontaneous magnetization. There are zero-energy modes for the orientation of the magnetization to be continuously changed forming spin waves. When the rotating angle depends on the space, i.e., the rotating modes has the finite wavenumber, the excitation energy is finite and converges to zero in the limit of the zero wavenumber. Such a mode converging to zero-energy mode is called NG mode. The characteristic of the NG mode depends on the spontaneously broken symmetry. When a stable topological defect exist, the system spontaneously loses its translational (or rotational) symmetry, and NG modes appears due to these symmetries and localizes on the topological defect. For the non-relativistic system (more precisely, Lorentz symmetry of the system is spontaneously broken), several NG modes sometimes couples to one NG mode. In the case of the Heisenberg model, we can naively consider two independent NG modes for the magnetization to change to the two direction perpendicular to the magnetization vector. However, these two NG modes couple to one NG mode forming a spiral spin wave. The coupling of several NG modes sometimes occur between the natural NG mode propagating the bulk system and the NG mode localized on the topological defect. A right movie shows the coupling of the bulk spin wave and the ripple wave localized on the magnetic domain wall forming a coupled NG mode realized in the Heisenberg model with a easy-axis interaction. |
A coupled NG mode between a spin wave (arrows) and a ripple wave localized on a magnetic domain wall (red surface). |
| The standard Heisenberg model can induce a tube-like topological defect called Skyrmion. There is a similar NG mode for the Skyrmion. The Skyrmion appeared in the Heisenberg model does not change the energy even when its thickness is changed. Namely, there is a NG mode called dilatation for changing the thickness of the Skyrmion. However, the dilatation cannot exist as a pure thickness-changing NG mode and couples to the bulk spin wave. | A coupled NG mode between the dilatation localized on the Skyrmion (gray surface) and the spin wave (arrows). |
Nucleations and decays of vortices through a rapid quench of the temperature.
|
We consider the dynamics for quenching a equilibrium state at the temperature much higher than the superfluid critical temperature to nearly zero temperature. The initial state has no macroscopic wave function. When the system cooled close to the critical temperature, the macroscopic wave function is partially nucleated and grows locally. The phase of the wave function depends on the space and highly randomized at first, and is gradually smoothed to form the unique value. However, several points can be continuously smoothed forming quantized vortex rings (called Kibble mechanism). Several rings are annihilated and several rings grow through reconnections, and finally, very long vortex loops are formed and decay very slowly. Because vortices cannot be continuously annihilated due to their topological stability, it takes very long for the system to be equilibrated. In other words, vortices nucleated through the rapid temperature quench prohibit equilibration Right movie shows the dynamics of vortices through the rapid temperature quench. In the first stage, point-like structures are formed, filling the whole system. They are small vortex loops nucleated by the failure for the macroscopic wave function to be smoothly connect (They are so small that loop structures of them cannot be seen). After a while, point-like structures are annihilated and we can see long string-like vortices. Alghouth, their lengths are decreased through reconnections, they survives very long. This dynamics is similar to quantum turbulence in regard to complicated vortex behavior, but obeys different but universal statistical low. There are still open questions remained such as the relationship between the dynamics and topological charges vortex. |
Dynamics of vortices nucleated through the rapid temperature quench. |